We at Calcblog hope you had a great winter vacation! So far, we’ve posted tutorials on graphing in rectangular/Cartesian and polar coordinates, but there are other ways to specify functions. One of these is as a function of a parameter, or a so called “parametric” equation.
This might be useful, for example, for writing X and Y coordinates of an object as a function of the parameter T, for time. We might have the following equations:
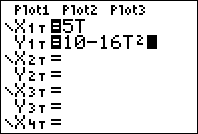
- X=5T
- Y=10-16T²
A glance into your physics textbook might tell you that these equations describe the coordinates of a particle with an X velocity of 5 feet per second, starting out 10 feet in the air and subject to Earth’s gravity. Let’s plot this on our calculator.
First, press to get to the mode menu, and use the arrow keys to select PAR. Press .
Second, press to get to the equation entry screen. Enter the corresponding X and Y equations.
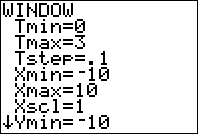
Third, press to get to the window screen. This is one of the most important parts of the process. In addition to setting the normal display limits, you must also specify a minimum T, a maximum T, and a step with which to plot the points. If you specify too large of a Tstep, your plot will look jagged or even misleading. If you specify one that is too small, it may take longer for your equation to graph.
Here, we’re sticking with the standard window (which you can always reach by pressing and selecting ZStandard), but setting 0≤T≤3 with a step of .1 seconds.
Press to view the plot. We see the path of our object plotted out in x-y coordinates.
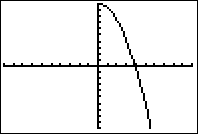
Press to use the left and right arrow keys to trace out the curve. It looks like the particle hits the “ground” (Y=0) at around .8 seconds. We can solve this exactly by solving 0=10-16T², or T=√(10/16), which is about .791 s.

Parametric equations can be a very practical way of looking at the world and are very useful in science, engineering, and design. We hope this tutorial was a useful introduction or refresher. As always, questions, comments, and suggestions are welcome via our contact form. Thank you for reading!
