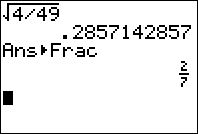
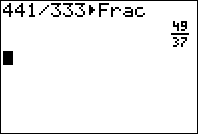
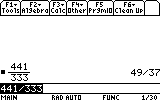
There are a lot of misconceptions about calculator use on the SAT. This article covers some basic strategies on how to use your calculator “smarter.” It also attempts to dispel some of the myths you’ve probably heard about calculators and test prep, such as the idea that a calculator won’t help you improve your math and test scores, (studies show this is false) or ideas about how useful calculator programs can be. Read on to learn more about how to get the most out of your SAT prep experience.
Calcblog Newswire
2014.01.05: Just posted a new tutorial on plotting parametric functions on the TI-83+ and TI-84+. Hope you find it useful! 2013.05.05: We posted a new video tutorial on using variables to perform calculations on your graphing calculator. Watch it! 2013.04.08: Check out our great new guide on learning the HP 50g and its RPN option. 2013.03.23: Just posted a quick guide to entering logarithms on your calculator.-
Recently Popular
- SAT Test Prep #1: Mean, Mode, and Median on the TI-83 Plus, TI-84 Plus, and TI-89
- How to Enter Logarithms on Your Graphing Calculator
- Calculating Derivatives on the TI-83 Plus, TI-84 Plus, TI-89, TI-92 Plus, and Voyage 200
- Business and Finance Math #2: Calculating the Effective Annual Rate (EAR) on Your TI BA II Plus or HP 12c
- Getting Started with the HP 50g
Calculators
- No categories
Categories
- No categories
Visit Our Sponsors